Question

What quadratic function does the graph represent?

Answer 1

`f(x)=-(1)/(3)(x-2)^2+4`,

Explanation:

The function of the graph can be written in the vertex form as

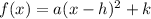`f(x)=a(x-h)^2+k`, where V(h,k)=V(2,4) is the vertex of the quadratic function.

We substitute the value to obtain;

`f(x)=a(x-2)^2+4`,

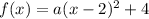The point (5,1) lies on the graph so we use it to determine the value of a.

`1=a(5-2)^2+4`,

`1-4=a(3)^2`,

`-3=9a`,

`a=-(1)/(3)`

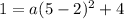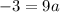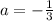
The required equation is

`f(x)=-(1)/(3)(x-2)^2+4`,