Question

Confused, I’d appreciate help!

Answer 1

The fundamental theorem of algebra says this cubic has three roots, so we could write it as

`4x^3+32x^2+79x+60=4(x-a)(x-b)(x-c)`

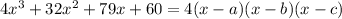
We're told one of the roots is equal to the sum of the other two, so we could take
`c=a+b`:

`4x^3+32x^2+79x+60=4(x-a)(x-b)(x-a-b)`

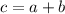If we expand the right side, we get

`4\bigg(x^3-2(a+b)x^2+(a^2+3ab+b^2)x-(a^2b+ab^2)\bigg)`

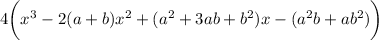
For two polynomial to be equal, the coefficients of terms of the same degree must match, so that

`\begin{cases}4=4&(x^3)\\32=-8(a+b)&(x^2)\\79=4(a^2+3ab+b^2)&(x)\\60=-4(a^2b+ab^2)&\text{(constant)}\end{cases}`

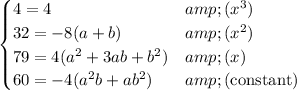
Now we can find
`a,b,c`.

`32=-8(a+b)=-8c\implies c=-4`

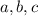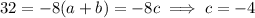
This tells us that
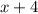`x+4` is a factor, so dividing the original cubic by this returns a remainder of 0.

`(4x^3+32x^2+79x+60)/(x+4)=4x^2+16x+15=4\left(x+\frac32\right)\left(x+\frac52\right)`

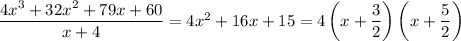
which further tells us that
`a=-\frac32` and
`b=-\frac52`.