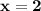Answer:
1)Solving the expression
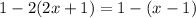 we get
we get

2) solving the equation
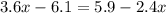 we get
we get
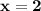
Explanation:
We need to solve the expressions:
1)
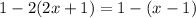
Solving
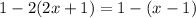
First solving the brackets

Simplifying

Adding 4x on both sides

Switching the sides
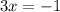
Divide both sides by 3
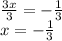
So, Solving the expression
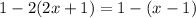 we get
we get

2)
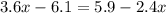
Solving:
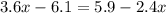
Adding 6.1 on both sides
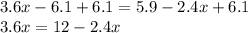
Adding 2.4x on both sides
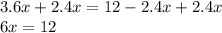
Divide both sides by 6
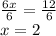
So, solving the equation
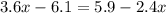 we get
we get