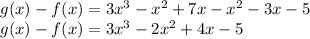Question

Can someone help me? Thank you so much!!!

Answer 1

Answer is C

Explanation:

F(x)=X^2+3x+5-G(x)= 3x^3-X^2+7x

(F-G(x))= -3x^3+2x^2-4x+5

Answer 2

Answer: OPTION D

Explanation:

Given the functions f(x) and g(x) shown in the figure attached, you have that g(x)-f(x) means that you must subtract both functions.

Therefore, keeping this on mind, you have:

`g(x)-f(x)=3x^(3)-x^(2)+7x-(x^(2)+3x+5)`

Now, you must distributive the negative sign that is in front of the parentheses and add the like terms. Then, you obtain:

`g(x)-f(x)=3x^(3)-x^(2)+7x-x^(2)-3x-5\\g(x)-f(x)=3x^(3)-2x^(2)+4x-5`