Question

From a window 20 feet above the ground, the angle of elevation to the top of a building across

the street is 78°, and the angle of depression to the base of the same building is 15°. Find the
height of the building across the street.

Answer 1

Answer: The answer is 381.85 feet.

Step-by-step explanation: Given that a window is 20 feet above the ground. From there, the angle of elevation to the top of a building across the street is 78°, and the angle of depression to the base of the same building is 15°. We are to calculate the height of the building across the street.

This situation is framed very nicely in the attached figure, where

BG = 20 feet, ∠AWB = 78°, ∠WAB = WBG = 15° and AH = height of the bulding across the street = ?

From the right-angled triangle WGB, we have

`(WG)/(WB)=\tan 15^\circ\\\\\\\Rightarrow (20)/(b)=\tan 15^\circ\\\\\\\Rightarrow b=(20)/(\tan 15^\circ),`

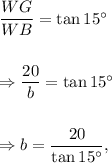
and from the right-angled triangle WAB, we have'

`(AB)/(WB)=\tan 78^\circ\\\\\\\Rightarrow (h)/(b)=\tan 15^\circ\\\\\\\Rightarrow h=\tan 78^\circ*(20)/(\tan 15^\circ)\\\\\\\Rightarrow h=361.85.`

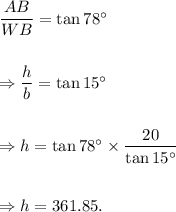
Therefore, AH = AB + BH = h + GB = 361.85+20 = 381.85 feet.

Thus, the height of the building across the street is 381.85 feet.