Answer:
18π ft2
Explanation:
The circle has a radius of 12 ft and the angle of the sector is 45°.
Area of the sector (A) is given by the formula below:
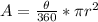
Where
 is the angle of the sector and r is the radius of the circle
is the angle of the sector and r is the radius of the circle
We know r = 12 and
 = 45°
= 45°
Plugging these into the formula and simplifying, we get:
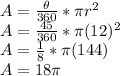
Thus, the area is
 sq. ft.
sq. ft.