Answer:
It will take 9 years for the population to double
Explanation:
Exponential Growth
The natural growth of some magnitudes can be modeled by the equation:
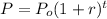
Where P is the actual amount of the magnitude, Po is its initial amount, r is the growth rate and t is the time.
The population of a city grows at a rate of r=8% = 0.08 per year. We are required to find when (t) the population will double, or P=2Po.
Substituting in the equation:
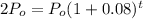
Simplifying:

Taking logarithms:

Applying the exponent property of logs:

Solving for t:
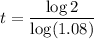
Calculating:
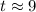
It will take 9 years for the population to double