There are infinite possible graphs for each of the questions. I am assuming that the purpose of this assignment is to get you to graph different types of equations and then transform them (by shifting to the left, right, up, down and/or stretching them) in order to see how the graphs move. I suggest you do this because it will be helpful for you to understand transformations.
Here are the answers and possible graphs. I have attached a snapshot of the maximum number of intersections for each graph.
a) They can intersect 0, 1, or 2 times
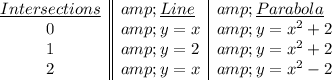
b) They can intersect 0, 1, or 2 times
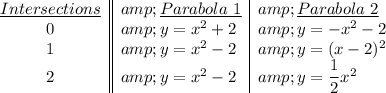
c) They can intersect 0, 1, 2, 3, or 4 times
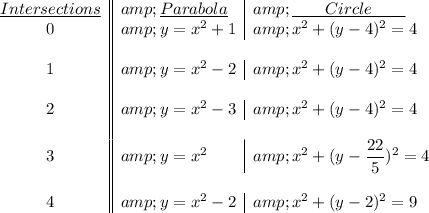
d) They can intersect 0, 1, 2, or 3 times
