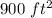Question

Tyler has 120 linear feet of fencing to put around his garden. If he uses the fence to border a rectangular garden, what is the maximum area his garden can be? Answer should have units written out such as yards, square centimeters or cubic inches.

Answer 1

The maximum area is
`900\ ft^(2)`

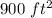
Explanation:

Let

x----> the length of rectangle

y---> the width of rectangle

we know that

The perimeter of rectangle is equal to

`P=2(x+y)`

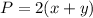
we have

`P=120\ ft`

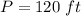
so

`120=2(x+y)`

`60=(x+y)`

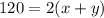
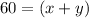
`y=60-x`------> equation A

Remember that

The area of rectangle is equal to

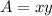`A=xy` -----> equation B

substitute equation A in equation B

`A=x(60-x)`

`A=-x^(2) +60x`

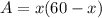
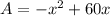
This is a vertical parabola open downward

The vertex is a maximum

The y-coordinate of the vertex of the graph is the maximum area of the garden and the x-coordinate is the length for the maximum area

using a graphing tool

The vertex is the point
`(30,900)`

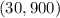
see the attached figure

Find the value of y

`y=60-x` ----->
`y=60-30=30\ ft`

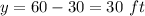
The dimensions of the rectangular garden is
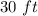`30\ ft` by
`30\ ft`

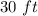
For a maximum area the garden is a square

The maximum area is
`900\ ft^(2)`