Question

The length of a rectangle was reduced by 10%

and the width by x%. If the resulting
dimensions reduced the area by 32.5%,
what is the value of x?

Answer 1

x = 25

Step-by-step explanation:

We can let an arbitrary rectangle has a length and width of 10, making the total area 10*10 or 100. We know that the length of the rectangle is reduced by 10%, meaning the new length is now 10 - (0.1*10), or 9. The actual width is unknown, but we do know that it will be an x% reduction of the original width: 10 - (0.01x*10), or 10 - 0.1x. We are also given that the area is reduced by 32.5%. This means the new area is 100 - (0.325*100), or 100 - 32.5, which is 67.5. Now, we can use the area formula (length*width) to find the value of x:

`length*width=area\\9*(10-0.1x)=67.5\\90-0.9x=67.5\\-0.9x=-22.5\\x=25`

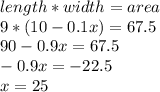
This means that the value of x is 25, or the width was reduced by 25%