Question

Identify the equation of the parent function y=x^3 that is horizontally streched by a factor of 1/5 and reflected over the y axis

Answer 1

`y=(-x^(3))/(125)`

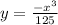
Explanation:

As we know that,

'Horizontal stretch, stretches the function left or right on the x-axis'.

The general form of horizontal stretch is given by 'f(kx)', where 0<k<1 is the factor by which function is stretched horizontally.

Since, the function
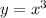`y=x^(3)` which is stretched by a factor of
`(1)/(5)`.

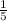The new function is
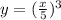`y=((x)/(5))^(3)` i.e.
`y=(x^(3))/(125)`.

Further, the function is reflected over y-axis.

That is, the reflected function is
`y=((-x)^(3))/(125)` i.e.
`y=(-x^(3))/(125)`.

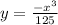Hence, the required function is
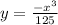`y=(-x^(3))/(125)`.