Question

34. For the following exercises, given each set of information, find a linear equation satisfying the conditions, if possible.

34. Passes through (-1, 4) and (5, 2)

Answer 1

The linear equation for the line which passes through the points given as (-1,4) and (5,2), is written in the point-slope form as
`$y=(1)/(3) x-(13)/(3)$`.

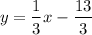Explanation:

A condition is given that a line passes through the points whose coordinates are (-1,4) and (5,2).

It is asked to find the linear equation which satisfies the given condition.

Step 1 of 3

Determine the slope of the line.

The points through which the line passes are given as (-1,4) and (5,2). Next, the formula for the slope is given as,

`$m=(y_(2)-y_(1))/(x_(2)-x_(1))$`

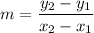
Substitute 2&4 for
`$y_(2)$` and
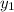`$y_(1)$` respectively, and
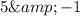`$5 \&-1$` for
`$x_(2)$` and
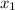`$x_(1)$` respectively in the above formula. Then simplify to get the slope as follows,

`m=(2-4)/(5-(-1))$\\ $m=(-2)/(6)$\\ $m=-(1)/(3)$`

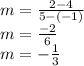
Step 2 of 3

Write the linear equation in point-slope form.

A linear equation in point slope form is given as,

`$y-y_(1)=m\left(x-x_(1)\right)$`

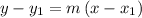
Substitute
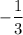`$-(1)/(3)$` for m,-1 for
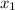`$x_(1)$`, and 4 for
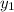`$y_(1)$` in the above equation and simplify using the distributive property as follows,

`y-4=-(1)/(3)(x-(-1))$\\ $y-4=-(1)/(3)(x+1)$\\ $y-4=-(1)/(3) x-(1)/(3)$`

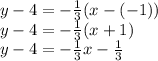
Step 3 of 3

Simplify the equation further.

Add 4 on each side of the equation
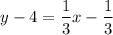`$y-4=(1)/(3) x-(1)/(3)$`, and simplify as follows,

`y-4+4=(1)/(3) x-(1)/(3)+4$\\ $y=(1)/(3) x-(1+12)/(3)$\\ $y=(1)/(3) x-(13)/(3)$`

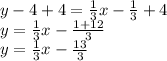
This is the required linear equation.