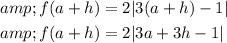Question

For the following exercises, evaluate the function at the indicated values:

f(−3); f (2); f(−a); −f(a); f (a + h).
6 f (x) = 2∣3 x − 1∣

Answer 1

The evaluated function for the indicated values is given below.

The value of f(-3) is 20 .

The value of f(2) is 10 .

The value of f(-a) is
`$2|-3 a-1|$`.

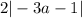The value of -f(a) is
`$-2|3 a-1|$`.

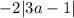The value of f(a+h) is
`$2|3 a+3 h-1|$`.

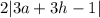Explanation:

A function
`$f(x)=2|3 x-1|$` is given.

It is required to evaluate the function at
`$f(-3) ; f(2) ; f(-a) ;-f(a) ; f(a+h)$`.

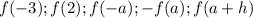To evaluate the function, substitute the indicated values in the given function to determine the output values and simplify the expression.

Step 1 of 5

The given function is
`$f(x)=2|3 x-1|$`.

To evaluate the function at f(-3), substitute -3 in the given function
`f(x)=2|3 x-1|$\\ $f(-3)=2|3(-3)-1|$\\ $f(-3)=2|-9-1|$\\ $f(-3)=2|-10|$\\ $f(-3)=2(10)$\\ $f(-3)=20$`

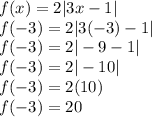
Step 2 of 5

To evaluate the function at $f(2)$, substitute 2 in the given function.

`f(x)=2|3 x-1|$\\ $f(2)=2|3(2)-1|$\\ $f(2)=2|6-1|$\\ $f(2)=2(5)$\\ $f(2)=10$`

Step 3 of 5

To evaluate the function at f(-a), substitute -a in the given function.

`$$\begin{aligned}&f(x)=2|3 x-1| \\&f(-a)=2|3(-a)-1| \\&f(-a)=2|-3 a-1|\end{aligned}$$`

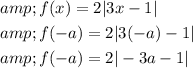
Step 4 of 5

To evaluate the function at -f(a), substitute a in the given function.

`f(x)=2|3 x-1|$\\ $-f(a)=-2|3(a)-1|$\\ $-f(a)=-2|3 a-1|$`

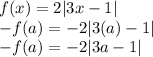
Step 5 of 5

To evaluate the function at f(a+h), substitute a+h in the given function.
`$f(x)=2|3 x-1|$`

`$$\begin{aligned}&f(a+h)=2|3(a+h)-1| \\&f(a+h)=2|3 a+3 h-1|\end{aligned}$$`