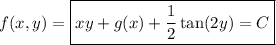1 Answer

Gstercken · Answer 1 · 2023-11-23T22:11:16+0000

Multiply both sides by
to get an exact equation.

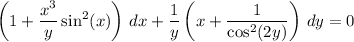
$\left(1 + \frac{x^3}y \sin^2(x)\right) \, dx + \frac1y \left(x + \frac1{\cos^2(2y)}\right) \, dy = 0$

$\implies \underbrace{(y + x^3 \sin^2(x))}_(M(x,y)) \, dx + \underbrace{(x + \sec^2(2y))}_(N(x,y)) \, dy = 0$

This ODE is exact since
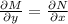$(\partial M)/(\partial y) = (\partial N)/(\partial x)$ . Then the solution is given by an implicit function

f(x,y) = C

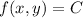
Taking differentials on both sides by the chain rule gives

$(\partial f)/(\partial x) \, dx + (\partial f)/(\partial y) \, dy = 0$

so that we have the system of partial differential equations

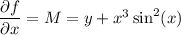
$(\partial f)/(\partial x) = M = y + x^3 \sin^2(x)$

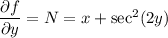
$(\partial f)/(\partial y) = N = x + \sec^2(2y)$

Integrate both sides of the first of these equations with respect to
to recover
.

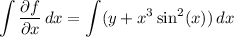
$\displaystyle \int (\partial f)/(\partial x) \, dx = \int (y + x^3 \sin^2(x)) \, dx$

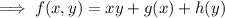
$\implies f(x,y) = xy + g(x) + h(y)$

where
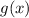g(x) is the antiderivative of
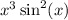$x^3\sin^2(x)$ (and is easy enough to compute by parts).

Differentiating both sides with respect to
gives

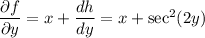
$(\partial f)/(\partial y) = x + (dh)/(dy) = x + \sec^2(2y)$

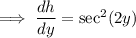
$\implies (dh)/(dy) = \sec^2(2y)$

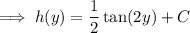
$\implies h(y) = \frac12 \tan(2y) + C$

Then the general solution to the ODE is

$f(x,y) = \boxed{xy + g(x) + \frac12 \tan(2y) = C}$

Please log in or register to add a comment.