Question

`\boxed{\bf{Help\;please}}`

____________________

`\boxed{\bf{Answer\;Requirements-:}}`
No spam
No absurd answers

`\bf{Only`
People who are good at trig

`\bf{should\;answer}`

Answer 1

-8m

Explanation:

This question only requires to to substitute t = 0 and t = 1 into the equation to find the displacement.

Lets find the displacement at t = 0.

`x(0)=4sin(\pi (0)+(\pi )/(2) )\\=4sin((\pi )/(2) )\\= 4m`

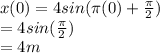
Lets find the displacement at t = 1.

`x(1) = 4sin(\pi (1)+(\pi )/(2) )\\=4sin(\pi +(\pi )/(2) )\\=-4m`

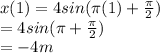
Total displacement = Final Position - Initial Position

= x(1)-x(0)

= -4m - 4m

= -8m