Answer:
The graph of pairwise function is shown below.
Explanation:
The given piece wise function is
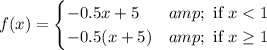
It means for x<1, the function is defined as
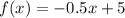
At x=0,
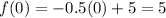
At x=-1,
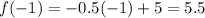
It means the graph passing through (0,5) and (-1,5.5). Joint these point to draw a line of x<1 and there is an open circle at x=1, because the the sign of inequality is <.
It means for x≥1, the function is defined as

At x=1,
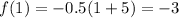
At x=2,
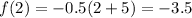
It means the graph passing through (1,-3) and (2,-3.5). Joint these point to draw a line of x≥1 and there is an closed circle at x=1, because the the sign of inequality is ≥.
The graph of pairwise function is shown below.