Question

Write the slope-intercept form of the equation with the given characteristics.

1. Slope = 1/4, y-intercept = 2

2. Slope = -3/2, passes through (-4,7)

3. Passes through (-2,1) and (2,-5)

4. Parallel to the line y= -3x+5, passes through (-4,5)

5. Perpendicular to the line y=1/2x-8, passes through (7,-6)

PLEASE ANSWER BECAUSE I'M STUDYING FOR FINALS ALONG WITH A BUNCH OF OTHER SUBJECTS

Answer 1

1. y=1/4x+2

2. y=-3/2x +1

3. y = -3/2x -2

4. y= -3x -49

5. y = -2x +8

Explanation:

1. Use slope intercept, y=mx+b where m =1/4 and b=2. y=1/4x+2

2. Use the point-slope form to write the equation, then simplify and convert into the slope intercept form.

(y-7)=-3/2(x--4)

y-7=-3/2(x+4)

y-7=-3/2x-6

y = -3/2x -6 +7

y=-3/2x +1

3. To write the equation of a line we must have a slope and a point. To find the slope we use the slope formula and substitute (x,y) points in it as shown below:

`m=(y_2-y_1)/(x_2-x_1)=(-5-1)/(2--2)=(-5+-1)/(2+2)=(-6)/(4)=(-3)/(2)`

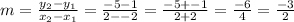
Now that we have the slope, plug in the slope and choose one point to plug into the point slope formula. Use the point-slope form to write the equation, then simplify and convert into the slope intercept form.

(y--5)=-3/2(x-2)

y+5=-3/2(x-2)

y+5=-3/2x + 3

y = -3/2x -2

4. Use the point-slope form to write the equation, then simplify and convert into the slope intercept form. The slope is -3 since parallel lines have the same slope.

(y--4)=-3(x-5)

y+4=-3 (x+15)

y+4=-3x -45

y= -3x -49

5. Use the point-slope form to write the equation, then simplify and convert into the slope intercept form. The slope is -2 since perpendicular lines have the negative reciprocal slopes. So 1/2 becomes -2.

(y--6)=-2(x-7)

y+6=-2 (x-7)

y+6 = -2x + 14

y = -2x +8