Question

Just an easy question for 100 Points!
Check my other easy 100 point questions :)

Answer 1

#1

y=x+5

• Shifted 5units left

#2

y=3/8

• Horizontally Streched by a factor 8/3 parallel to x axis

#3

• y=-x-2

Reflection by y axis as x will be negative and shifting 2 units right

#4

y=6x+1

• vertically streched by a factor 6 and shifting 1 units left

#5

y=x-11

• Shifting 11 units left

#6

y=8x

Horizontally streched by 1/8 factor

Answer 2

1. Translated 5 units left.

2. Vertical stretch by a factor of 3/8.

3. Reflection in the x-axis, then translated 2 units down.

4. Vertical stretch by a factor of 6, then translated 1 unit up.

5. Translated 11 units down.

6. Vertical stretch by a factor of 8.

7. Vertical stretch by a factor of 1/3, then reflection in the x-axis.

Explanation:

Transformations

For a > 0

`f(x+a) \implies f(x) \: \textsf{translated $a$ units left}.`

`f(x-a) \implies f(x) \: \textsf{translated $a$ units right}.`

`f(x)+a \implies f(x) \: \textsf{translated $a$ units up}.`

`f(x)-a \implies f(x) \: \textsf{translated $a$ units down}.`

`a\:f(x) \implies f(x) \: \textsf{stretched parallel to the $y$-axis (vertically) by a factor of $a$}.`

`f(ax) \implies f(x) \: \textsf{stretched parallel to the $x$-axis (horizontally) by a factor of $(1)/(a)$}.`

`-f(x) \implies f(x) \: \textsf{reflected in the $x$-axis}.`

`f(-x) \implies f(x) \: \textsf{reflected in the $y$-axis}.`

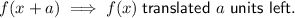
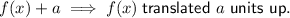
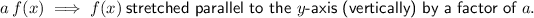
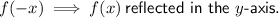
Parent function:

`y=x`

`\textsf{1.} \quad y=x+5`

Translated 5 units left.

`\textsf{2.} \quad y=(3)/(8)x`

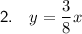
Vertical stretch by a factor of 3/8.

`\textsf{3.} \quad y=-x-2`

Reflection in the x-axis, then translated 2 units down.

`\textsf{4.} \quad y=6x+1`

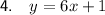
Vertical stretch by a factor of 6, then translated 1 unit up.

`\textsf{5.} \quad y=x-11`

Translated 11 units down.

`\textsf{6.} \quad y=8x`

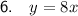
Vertical stretch by a factor of 8.

`\textsf{7.} \quad y=-(1)/(3)x`

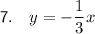
Vertical stretch by a factor of 1/3, then a reflection in the x-axis.