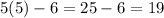Answer:
The length of FG is
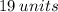 .
.
Explanation:
Since the altitude of the triangle bisects the third side, triangle FGH is isosceles.
Therefore
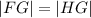
This implies that,
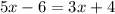
This is a linear equation where we easily solve for
 .
.
We group like terms to get,
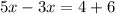

We divide both sides by 2 to obtain,
 .
.
The length of FG is