Question

The graph of f(x) = x2 has been shifted into the form f(x) = (x − h)2 + k:

What is the value of k?

−3
−2
2
3

Answer 1

k = 3

Explanation:

In (x - h)^2 + k, k determines whether the graph moves up or down (vertical movements). Therefore to see what the value of k is, look at the graph and where the y-coordinate is.

The y-coordinate is 3, so the value of k is 3 (k moved the graph 3 units up from 0).

Answer 2

Final answer is 3.

Explanation:

Question says that the graph of
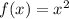`f(x)=x^2` has been shifted into the form
`f(x)=(x-h)^2+k`.

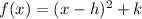Now we need to find about what is the value of k.

We know that
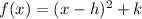`f(x)=(x-h)^2+k` formula is vertex form of parabola where (h,k) gives vertex of the parabola.

From graph, we see that vertex of the parabola is at point (2,3).

Now compare (h,k) with (2,3).

We get: k=3.

Hence final answer is 3.