Question

Consider the function f(x)= (x+4)(x-2) what is the equation of the axis of symmetry

Answer 1

if we zero out f(x), namely make y = 0, we can get the roots or x-intercepts for this quadratic equation

`\bf 0 = (x+4)(x-2)\implies \begin{cases} 0=x+4\implies &-4=x\\ 0=x-2\implies &2=x \end{cases}`

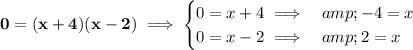
now, the equation is in x-terms, meaning is a vertically opening parabola, so the axis of symmetry will be x = something, a vertical line.

well, we have two x-intercepts, one at -4 and another at 2, and the vertex is right half-way between those guys

-4------------(-1)------------2

so the vertex is at x=-1, namely the axis of symmetry is x = -1.