Question

A prop for a school play is a sphere that will be hung from the ceiling. The surface area of the sphere is 5 024 cm'. If the surface area of the sphere can be found by using the formula SA = 4πr^2. where r is the radius, what is the radius of the sphere, to the nearest cm?

Answer 1

The radius of the sphere, to the nearest cm:

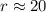`r\approx 20` cm

Explanation:

The surface area of a sphere is given by the formula

A = 4πr²

where r is the radius of the sphere.

Given

• The surface area of sphere A = 5024 cm²

The radius of the sphere can be determined such as

`A\:=\:4\pi r^2`

`r\:=\:(1)/(2)\sqrt{(A)/(\pi )}`

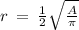
Plug in Surface Area of sphere = 5024, π = 3.14 in the formula

`\:r\:=\:(1)/(2)\sqrt{(5024)/(3.14)}\:\:`

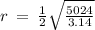
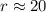`r\approx 20` cm

Therefore, the radius of the sphere, to the nearest cm:

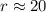`r\approx 20` cm