Answer:
Please check the explanation.
Explanation:
Part a)
Given the table
Year Value ($)
1 18,000
2 15,300
3 13,005
Let us check the sequence
18000, 15300, 13005,...
Let us find the common ratio 'r' of all the adjacent terms to check whether the sequence represents the geometric sequence or not, using the formula such as:
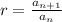

As the ratio of all the adjacent terms is the same, so
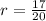
Therefore, the given sequence is a geometric sequence
Part b)
Given the sequence
18000, 15300, 13005,...
The nth term of the geometric sequence is

here
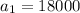
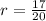
so
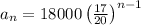
In order to determine the car worth in the 15th year, substitute n = 15
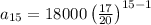
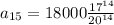

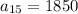 $
$
Therefore, the worth of a car in the 15th year will be: $1850