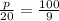Explanation:
If a variables varies jointly, we can just divide it by the other variables in relation to it.
For example, since p variables jointly as q and square of r, then
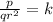
where k is a constant
First, let find k. Substitute p= 200
q= 2, and r=3.
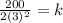
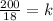
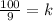
Now, since we know our constant, let find p.
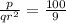
Q is 5, and r is 2.