Answer:
The probability of obtaining the letter p twice is 1/121
Explanation:
Probability of Recurring Events
There are 11 letters in the word 'independent', one of which is the letter 'p'.
When those letters are written on individual cards and they are put into a box, there are 11 different choices to pick at random.
This means the individual probability of getting a 'p' is:
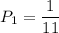
The card is reinserted into the box, leaving the sample space unaltered, thus the second card has the same probability:
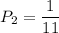
We'll use the multiplication rule. Just multiply the probability of the first event by the second.
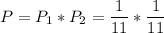
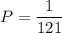
The probability of obtaining the letter p twice is 1/121