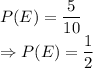Question

Adam has 10 blocks numbered 1 through 10. Which describes the probability of randomly choosing a block that has an even number?

Answer 1

`(1)/(2)`

Explanation:

Given that:

10 number of blocks which have numbers from 1 through 10.

To find:

Probability of randomly choosing a block that has an even number.

Solution:

The given set of numbers is: {1, 2, 3, 4, 5, 6, 7, 8, 9, 10}

There are a total of 5 Even numbers are {2, 4, 6, 8, 10}.

And There are a total of 5 Odd number are {1, 3, 5, 7, 9}.

Formula for probability of an event E can be observed as:

`P(E) = \frac{\text{Number of favorable cases}}{\text {Total number of cases}}`

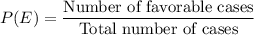
Here, Event E is randomly choosing an even number.

Number of favorable cases = 5

Total number of cases = 10

Therefore, the required probability is:

`P(E) = (5)/(10)\\\Rightarrow P(E) = (1)/(2)`