Question

Solve for y.
frankly im confusion
a:4.53
b.4
c.7.47
c.8

Answer 1

the answer to this is answer C:8.

Answer 2

8

Explanation:

Due to lines r & s being parallel to each other, we can apply the rule of alternate interior angles to solve for y in this problem.

Applying this, we'll be obtaining the equation that of:

`116=15y-4`

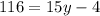
We now simply solve for y.

`120=15y\\y=8`

Therefore, y is equal to 8.

This can be checked by plugging it into the equation:

`15(8)-4`

`120-4`

`116`

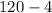
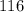
And look at that, we got 116 for our answer, exactly the same as the other angle.

Any questions feel free to ask :)