Question

A friend recently planned a camping trip. He had two flashlights, one that required a single 6-V battery and another that used two size-D batteries. He had previously packed two 6-V and four size-D batteries in his camper. Suppose the probability that any particular battery works is p and that batteries work or fail independently of one another. Our friend wants to take just one flashlight. For what values of p should he take the 6-V flashlight

Answer 1

The values of p = 0 < p ≤ 2/3

Explanation:

First we write the probability of 6V flashlight working representing it as a binomial mass function of a binomial random variable

P ( 6v flashlight working ) = P ( at least one 6V battery works )

= P ( 1 6v battery work ) + P ( 2 6v battery work )

= b( 2; 2, p ) + b( 1; 2, p )

write out a formula of b( 2; 2, p ) + b( 1; 2, p )

P ( 6v flashlight working ) = p^2 + 2p( 1 - p )

Next we write the probability of D flashlight working representing it as a binomial mass function of a binomial random variable

P ( D flashlight works ) = p ( at least two D batteries works )

= b( 4; 4, p ) + b(3;4, p ) + b(2; 4, p )

write out a formula of b( 4; 4, p ) + b(3;4, p ) + b(2; 4, p )

P ( D flashlight works ) =
`P^4 + 4p^3 ( 1- p ) + 6p^2 ( 1- p)^2`

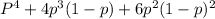
attached below is the remaining solution