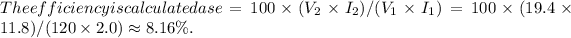Question

Part A: The primary coil of a transformer contains 100 turns; the secondary has 200 turns. The primary coil is connected to a size-AA battery that supplies a constant voltage of 1.5 volts. What voltage

asked Dec 17, 2021 35.1k views

Part A:

The primary coil of a transformer contains 100 turns; the secondary has 200 turns. The primary coil is connected to a size-AA battery that supplies a constant voltage of 1.5 volts. What voltage would be measured across the secondary coil?
Part B:
A transformer is intended to decrease the value of the alternating voltage from 500 volts to 25 volts. The primary coil contains 200 turns. Find the necessary number of turns N2 in the secondary coil.
Part C:
A transformer is intended to decrease the value of the alternating current from 500 amperes to 25 amperes. The primary coil contains 200 turns. Find the necessary number of turns N2 in the secondary coil.
Part D:
In a transformer, the primary coil contains 400 turns, and the secondary coil contains 80 turns. If the primary current is 2.5 amperes, what is the secondary current I2?
Part E:
The primary coil of a transformer has 200 turns and the secondary coil has 800 turns. The power supplied to the primary coil is 400 watts. What is the power generated in the secondary coil if it is terminated by a 20-ohm resistor?
Part F:
A transformer supplies 60 watts of power to a device that is rated at 20 volts. The primary coil is connected to a 120-volt ac source. What is the current I1 in the primary coil?
Part G:
The voltage and the current in the primary coil of a nonideal transformer are 120 volts and 2.0 amperes. The voltage and the current in the secondary coil are 19.4 volts and 11.8 amperes. What is the efficiency e of the transformer? The efficiency of a transformer is defined as the ratio of the output power to the input power, expressed as a percentage: e=100Pout/Pin.

Kracekumar asked

by Kracekumar

4.6k points

2 Answers

Ask a Question

Neil VanLandingham · Answer 1 · 2021-12-18T05:36:10+0000

Answer:

a) 0 V

b) 10 turns

c) 4000 turns

d) 12.5 A

e) 400 W

f) 0.5 A

g) 95.4%

Step-by-step explanation:

A

0

B

To solve this, we would be using the simple relationship between voltage and number of turns

V1/V2 = N1/N2

500/25 = 200/N2

20 = 200/N2

N2 = 200/20

N2 = 10 turns

C

Here also, we would be using the relationship between current and the number of turns

I1/I2 = N2/N1

500/25 = N2/20

20 = N2/20

N2 = 20 * 20

N2 = 4000 turns

D

Like in the previous question, current and the number of turn relationship is used

N1/N2 = I2/I1

400/80 = I2/2.5

5 = I2/2.5

I2 = 5 * 2.5

I2 = 12.5 A

E

The power remains unchanged at 400 W

F

Power = Voltage * Current

P = VI

I = P/V

I = 60/120

I = 0.5 A

G

95.4%

Josh Silveous · Answer 2 · 2021-12-21T13:14:11+0000

Part A: The voltage across the secondary coil is 3 V.

Part B: With a turns ratio of 10, the secondary coil has 10 turns.

Part C: The secondary coil, with 10 turns, decreases the current from 500 A to 25 A.

Part D: The secondary current is 12.5 A.

Part E: The power in the secondary coil is 1600 W.

Part F: The current in the primary coil is 0.5 A.

Part G:The efficiency of the transformer is approximately 8.16%.

Part A:

The voltage across the secondary coil
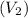$($V_2$)$ is determined by the turns ratio in a transformer, expressed as
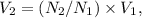$$V_2 = (N_2/N_1) * V_1$,$ where
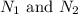$N_1\) and $N_2$ are the number of turns in the primary and secondary coils, respectively, and
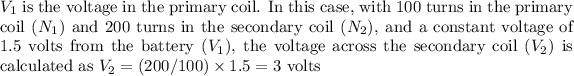$\(V_1$ is the voltage in the primary coil. In this case, with 100 turns in the primary coil ($N_1$) and 200 turns in the secondary coil ($N_2$), and a constant voltage of 1.5 volts from the battery ($V_1$), the voltage across the secondary coil ($V_2$) is calculated as $V_2 = (200/100) * 1.5 = 3$ volts$ .

Part B:

For a transformer intended to reduce the alternating voltage from 500 volts to 25 volts, the turns ratio
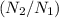$($N_2/N_1$)$ is equal to the voltage ratio (\
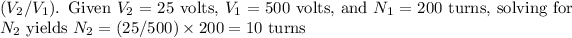$(V_2/V_1\)). Given $V_2 = 25$ volts, $V_1 = 500$ volts, and $N_1 = 200$ turns, solving for $N_2$ yields $N_2 = (25/500) * 200 = 10$ turns$ in the secondary coil.

Part C:

In transforming the alternating current from 500 amperes to 25 amperes, the turns ratio is given by
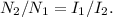$$N_2/N_1 = I_1/I_2$.$ With
$I_1 = 500$ amperes,
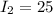$I_2 = 25$ amperes, and
$N_1 = 200$ turns, solving for
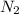$N_2$ results in
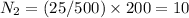$N_2 = (25/500) * 200 = 10$ turns in the secondary coil.

Part D:

The relationship between the primary current
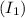$($I_1$)$ and the secondary
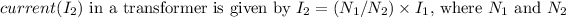$current ($I_2$) in a transformer is given by $I_2 = (N_1/N_2) * I_1$, where $N_1$ and $N_2$$ are the number of turns in the primary and secondary coils, respectively. For a transformer with 400 turns in the primary coil
$($N_1$)$ and 80 turns in the secondary coil
$($N_2$)$ , and a primary current
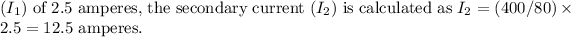
$($I_1$) of 2.5 amperes, the secondary current ($I_2$) is calculated as $I_2 = (400/80) * 2.5 = 12.5$ amperes.$

Part E:

The power
$($P_{\text{out}}$)$ generated in the secondary coil of a transformer can be determined by the turns ratio squared, multiplied by the power supplied to the primary coil
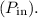$($P_{\text{in}}$).$ The formula is
$$P_{\text{out}} = (N_2/N_1)^2 * P_{\text{in}}$.$ With 200 turns in the primary coil
$($N_1$),$ 800 turns in the secondary coil
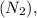$($N_2$),$ and 400 watts of power supplied to the primary coil
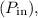$($P_{\text{in}}$),$ the power generated in the secondary coil is calculated as
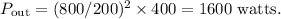
$$P_{\text{out}} = (800/200)^2 * 400 = 1600$ watts.$

Part F:

In a transformer, the primary current
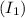$($I_1$)$ can be determined using the power formula
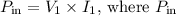$P_{\text{in}} = V_1 * I_1\), where $P_{\text{in}}$ is the power supplied to the transformer,
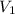$V_1$ is the voltage in the primary coil, and
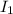$I_1$ is the current in the primary coil. Given that the power supplied is 60 watts
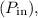$(\(P_{\text{in}}$),$ the voltage in the primary coil
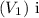$($V_1$) i$ s 120 volts, and solving for
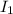$I_1$ gives
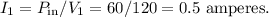
$$I_1 = P_{\text{in}}/V_1 = 60/120 = 0.5$ amperes.$

Part G:

The efficiency
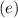$($e$)$ of a transformer is defined as the ratio of the output power
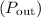$($P_{\text{out}}$)$ to the input power
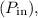$($P_{\text{in}}$),$ expressed as a percentage
$($e = 100 * P_{\text{out}}/P_{\text{in}}$).$ For a nonideal transformer, the given values are: voltage and current in the primary coil
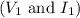$($V_1$ and $I_1$)$ are 120 volts and 2.0 amperes, respectively; voltage and current in the secondary coil
$($V_2$ and $I_2$)$ are 19.4 volts and 11.8 amperes, respectively.
$The efficiency is calculated as $e = 100 * (V_2 * I_2)/(V_1 * I_1) = 100 * (19.4 * 11.8)/(120 * 2.0) \approx 8.16\%$.$

0 Comments

Please log in or register to add a comment.

Please log in or register to answer this question.

2 Answers

0 Comments

Please log in or register to add a comment.

0 Comments

Please log in or register to add a comment.

Other Questions