Question

If f(x)=3x^5+2x^4+5, then what is the remainder when f(x) is divided by x−1?

Answer 1

The remainder is 10

Explanation:

The find the remainder when f(x) divided by g(x), find the value of x when g(x) = 0, then substitute it in f to find the value of the remainder

∵ f(x) = 3
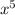`x^(5)` + 2
`x^(4)` + 5

∵ f(x) is divided by x - 1

∴ g(x) = x - 1

→ Equate g(x) by 0 to find x

∵ g(x) = 0

∴ x - 1 = 0

→ Add 1 to both sides

∵ x - 1 + 1 = 0 + 1

∴ x = 1

→ Substitute x in f by 1

∵ f(1) = 3
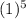`(1)^(5)` + 2
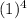`(1)^(4)` + 5

∴ f(1) = 3(1) + 2(1) + 5

∴ f(1) = 3 + 2 + 5

∴ f(1) = 10

∵ The remainder is the value of f(1)

∴ The remainder is 10