Question

the ratio of the measures of the sides of a triangle is 2:10:7 find the length of each side of the perimeter of the triangle is 228 meters

Answer 1

Length of side 1 = 24 m

Length of side 2 = 120 m

Length of side 3 = 84 m

Explanation:

The ratio of each side of triangle is: 2:10:7

Perimeter of triangle = 228 meters

We need to find the length of each side.

Let the common ratio be x

We can write the ratios as: 2x,10x and 7x

Length of side 1 = 2x

Length of side 2 = 10x

Length of side 3 = 7x

Using the formula:
`Perimeter\:of\:triangle=Sum\:of\:all\:sides\:of\:triangle\\`

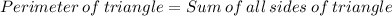
Putting values and finding x:

`Perimeter\:of\:triangle=Sum\:of\:all\:sides\:of\:triangle\\\\228=2x+10x+7x\\19x=228\\x=(228)/(19)\\x=12`

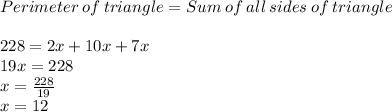
So, we get value of x=12

Now, finding length of each side by putting x=12:

Length of side 1 = 2x =2(12) = 24

Length of side 2 = 10x = 10(12) = 120

Length of side 3 = 7x = 7(12) = 84

So,

Length of side 1 = 24 m

Length of side 2 = 120 m

Length of side 3 = 84 m