Question

The gross weekly sales at a certain super market are a Gaussian random with mean $2200 and standard deviation $230. Assume that the sales from week to week are independent.

A) Find the probability that the gross sales over the next two weeks exceed $5000.
B) Find the probability that the gross weekly sales exceed $2000 in at least 2 of the next 3 weeks.

Answer 1

A) P(Z > 5000) = 0.0322

B) P( Y = 2 or 3) ≅ 0.9032

Explanation:

From the given information;

Suppose the sales for the first week are denoted by X and the sales for the second week are denoted by Y.

Then;

X & Y are independent and they follow a normal distribution.

i.e.

`XY \sim N(\mu,\sigma^2)`

`XY \sim N(2200,230^2)`

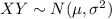
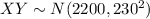
If we set Z to be equal to X+Y

Then,
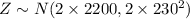`Z \sim N(2 * 2200,2 * 230^2)` since two normal distribution appears normal

`Z \sim N(4400,105800)`

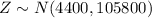
So;

`P(Z > 5000) = 1 - P( Z< (x = \mu)/(√(\sigma)))`

`P(Z > 5000) = 1 - P( Z< (5000-4400)/(√(105800)))`

`P(Z > 5000) = 1 - P( Z< (600)/(325.2691))`

`P(Z > 5000) = 1 - P( Z< 1.844626495)`

`P(Z > 5000) = 1 - P( Z< 1.85)`

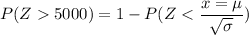
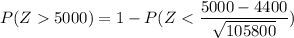
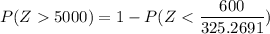
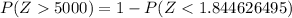
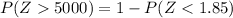
From the Z - tables;

P(Z > 5000) = 1 - 0.9678

P(Z > 5000) = 0.0322

B)

Let Y be the random variable that obeys the Binomial distribution.

Y represents the numbers of weeks in the next 3 weeks where the gross weekly sales exceed $2000

Thus;

`Y \sim Bin(3,p)`

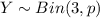
where;

`p = 1 - P( Z < (2000-2200)/(230))`

`p = 1 - P( Z < (-200)/(230))`

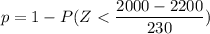
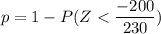
p = 1 - P( Z < - 0.869565)

From the Z - tables;

p = 1 - 0.1924

p = 0.8076

Now;

P(Y ≥ 2) = P(Y = 2) + P( Y =3 )

Using the formula

`P(X = r ) = ^nC_r * p^r * q ^(n-r)`

`P( Y = 2 \ or \ 3) =^ 3C_2 * 0.8076^2 * ( 1- 0.8076) ^ {3-2} + ^ 3C_3 * 0.8076^3 * ( 1- 0.8076) ^ {3-3}`

`P( Y = 2 \ or \ 3) =(3!)/(2!(3-2)!) * 0.8076^2 * ( 0.1924) ^ 1 + (3!)/(3!(3-3)!)* 0.8076^3 * ( 0.1924) ^ {0}`

`P( Y = 2 \ or \ 3) =0.3764600911 +0.526731063`

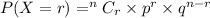
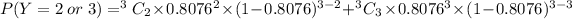
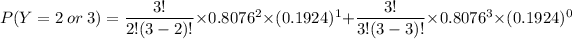
P( Y = 2 or 3) = 0.9031911541

P( Y = 2 or 3) ≅ 0.9032