Question

I will give lots of points please help

Answer 1

a) 81π in³

b) 27 in³

c) divide the volume of the slice of cake by the volume of the whole cake

d) 10.6%

e) see explanation

Explanation:

Part (a)

The cake can be modeled as a cylinder with:

• diameter = 9 in
• height = 4 in

`\sf Radius=(1)/(2)diameter \implies r=4.5\:in`

`\textsf{Volume of a cylinder}=\sf \pi r^2 h \quad\textsf{(where r is the radius and h is the height)}`

`\begin{aligned}\sf \implies \textsf{Volume of the cake} & =\pi (4.5)^2(4)\\ & = \sf \pi (20.25)(4)\\ & = \sf81 \pi \:\: in^3\end{aligned}`

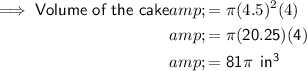
Part (b)

`\begin{aligned}\textsf{Circumference of the cake} & = \sf \pi d\\& = \sf 9 \pi \:\:in\end{aligned}`

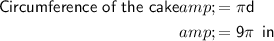
If each slice of cake has an arc length of 3 in, then the volume of each slice is 3/9π of the entire volume of the cake.

`\begin{aligned}\implies \textsf{Volume of slice of cake} & = \sf (3)/(9 \pi) * \textsf{volume of cake}\\\\& = \sf (3)/(9 \pi) * 81 \pi\\\\& = \sf (243 \pi)/(9 \pi)\\\\& = \sf 27\:\:in^3\end{aligned}`

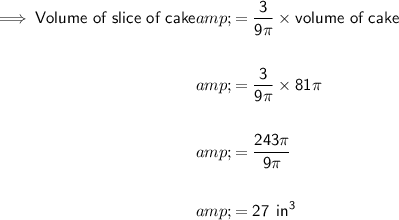
Part (c)

The volume of each slice of cake is 27 in³.

The volume of the whole cake is 81π in³.

To calculate the probability that the first slice of cake will have the marble, divide the volume of a slice by the volume of the whole cake:

`\begin{aligned}\implies \sf Probability & = \sf (27)/(81 \pi)\\\\& = \sf 0.1061032954...\\\\ & = \sf 10.6\% \:\:(1\:d.p.)\end{aligned}`

Part (d)

Probability is approximately 10.6% (see above for calculation)

Part (e)

If the four slices of cake are cut and passed out before anyone eats or looks for the marble, the probability of getting the marble is the same for everyone. If one slice of cake is cut and checked for the marble before the next slice is cut, the probability will increase as the volume of the entire cake decreases, until the marble is found. So it depends upon how the cake is cut and distributed as to whether Hattie's strategy makes sense.