Answer:
So the second option is the answer: Makayla invests her money 2 years longer.
Explanation:
Formula for compound interest:

Calvin:
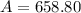
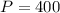
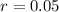

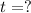
Makayla:
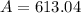

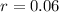
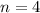
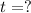
Lets solve for
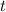 .
.
1) Divide both sides of the equation by
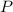 .
.
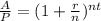
2) Take the natural log of both sides.
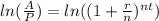
3) Rewrite the right side of the equation using properties of exponents.
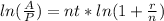
4) Divide each side of the equation by
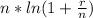
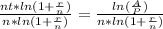
5) Cancel the common factor
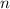 on the left side of the equation.
on the left side of the equation.
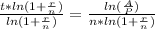
6) Cancel the common factor of
 .
.
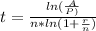
Now we have an equation for
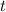 that we can use to answer your question.
that we can use to answer your question.
For Calvin:


For Makayla:
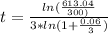

So the second option is the answer: Makayla invests her money 2 years longer.
Note: This question took me 2 minutes to answer but typing it took 30. lol