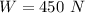Question

A lever is 3.2 m long. The distance from the fulcrum to the underside of a boulder to be lifted is 1.4 m. If a farmer pushes on the opposite end with 350 N, what is the maximum force that is applied to the boulder

Answer 1

The value is
`W = 450 \ N`

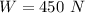
Step-by-step explanation:

From the question we are told that

The force applied at the other end is
`F = 350 \ N`

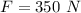
The length of the lever is
`l = 3.2 \ m`

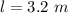
The distance from the fulcrum to the underside of a boulder is
`x_1 = 1.4 \ m`

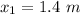
The distance from the fulcrum to the point where the farmer applied the force is

`x_2 = 3.2 - 1.4`

=>
`x_2 = 1.8 \ m`

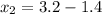
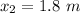
Generally at equilibrium the net torque experienced by the lever is 0 and this is mathematically represented as

`F * x_2 - W * x_1 = 0`

Here W is the maximum force applied to the boulder by the lever

So

`F * x_2 - W * x_1 = 0`

=>
`350 * 1.8- W * 1.4 = 0`

=>
`W = 450 \ N`